Displacement is a vector quantity, commonly denoted by the vector s, that reflects an object’s change in spatial position. The displacement of an object that moves from point A to point B is a vector whose tail is at A and whose tip is at B. Displacement deals only with the separation between points A and B, and not with the path the object followed between points A and B. By contrast, thedistance that the object travels is equal to the length of path AB.

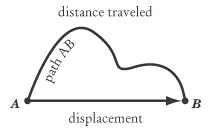
　　Students often mistake displacement for distance, and SAT II Physics may well call for you to distinguish between the two. A question favored by test makers everywhere is to ask the displacement of an athlete who has run a lap on a 400-meter track. The answer, of course, is zero: after running a lap, the athlete is back where he or she started. The distance traveled by the athlete, and not the displacement, is 400 meters.　　Example

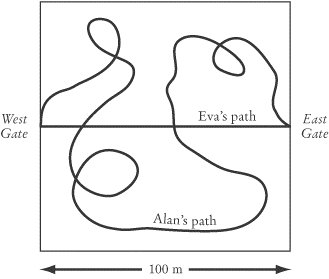
　　Alan and Eva are walking through a beautiful garden. Because Eva is very worried about the upcoming SAT II Physics Test, she takes no time to smell the flowers and instead walks on a straight path from the west garden gate to the east gate, a distance of 100 meters. Alan, unconcerned about the test, meanders off the straight path to smell all the flowers in sight. When Alan and Eva meet at the east gate, who has walked a greater distance? What are their displacements?

　　Since Eva took the direct path between the west and east garden gates and Alan took an indirect path, Alan has traveled a much greater distance than Eva. Yet, as we have discussed, displacement is a vector quantity that measures the distance separating the starting point from the ending point: the path taken between the two points is irrelevant. So Alan and Eva both have the same displacement: 100 meters east of the west gate. Note that, because displacement is a vector quantity, it is not enough to say that the displacement is 100 meters: you must also state the direction of that displacement. The distance that Eva has traveled is exactly equal to the magnitude of her displacement: 100 meters.

　　After reaching the east gate, Eva and Alan notice that the gate is locked, so they must turn around and exit the garden through the west gate. On the return trip, Alan again wanders off to smell the flowers, and Eva travels the path directly between the gates. At the center of the garden, Eva stops to throw a penny into a fountain. At this point, what is her displacement from her starting point at the west gate?

　　Eva is now 50 meters from the west gate, so her displacement is 50 meters, even though she has traveled a total distance of 150 meters.

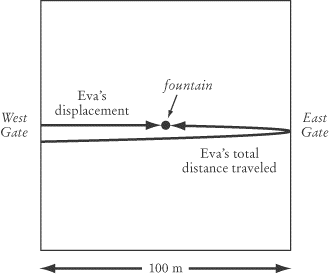
　　When Alan and Eva reconvene at the west gate, their displacements are both zero, as they both began and ended their garden journey at the west gate. The moral of the story? Always take time to smell the flowers!